Answer:
Intial Value: f(t) = 2 , Exponential Growth
Explanation:
To find the initial value, all we have to do is find the value of f(t) at t = 0
In this case the given equation becomes
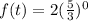
from the law of indices we know that any number with the power 0 is equal to 1 (except 0 with the power 0)
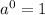
hence the above equation becomes
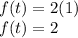
so the initial value is 2.
To find out whether this is exponential growth or exponential decay we need to see whether the base value of the power t is less than 1 or greater than 1, i.e. from
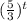
is
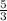 > 1 or
> 1 or
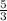 < 1
< 1
if the value is greater, then with each increment in power, the total value will increase while if it is less than 1 then with each increment in power the total value will decrease.
Hence since
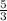 > 1 then this is an exponential growth
> 1 then this is an exponential growth