Answer:
Initial Value is
 , This function follows exponential decay
, This function follows exponential decay
Explanation:
The initial value of any time based function is the value of f(t) at t = 0. For calculating the initial value of this function all we have to do is insert 0 as the value of t.
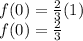
Hence the initial value is

To find out whether this function is exponential growth or decay we need to ascertain whether the base value of the power t is greater than or lesser than 1
In this case the base value of
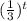 is
is
 which is lesser than 1, hence this function is exponential decay since with each increase in power the total value will decrease,i.e.
which is lesser than 1, hence this function is exponential decay since with each increase in power the total value will decrease,i.e.
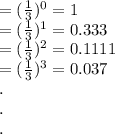
This can also be proven from the graph below