Question

A farmer has $1500 available to build an E-shaped fence along a straight river so as to create two identical pastures. The materials for the side parallel to the river cost $6 per foot, and the materials for the three sections perpendicular to the river cost $5 per foot. Find the dimensions for which the total area is as large as possible.

Answer 1

Answer: The largest dimensions that are possible would be 50 foot by 125 foot.

Explanation:

Since we have given that

Amount a farmer has available = $1500

Let x be the side perpendicular to the river.

Let y be the side parallel to the river.

Number of section perpendicular to the river = 3

cost of material for the side parallel to the river = $6 per foot

Cost of material for the side perpendicular to the river = $5 per foot

So, total cost becomes

`Cost=6y+5(3x)=1500\\\\Cost=6y+15x=1500\\\\y=(1500-15x)/(6)}`

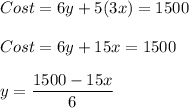
Area would be

`A=x* y\\\\A=x((1500-15x)/(6))\\\\A=(1)/(6)(1500x-15x^2)\\\\A'=(1)/(6)(1500-30x)`

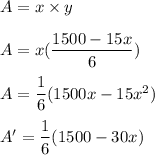
Now, put A' = 0 to get the critical points.

So, it becomes,

`(1)/(6)(1500-30x)=0\\\\1500-30x=0\\\\1500=30x\\\\x=(1500)/(30)\\\\x=50`

`A''=(-30)/(6)=-5<0`

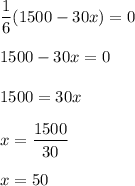
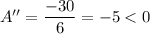
so, at x= 50 it will give maximum dimensions.

`y=(1500-15x)/(6)=(1500-15* 50)/(6)=(750)/(6)=125`

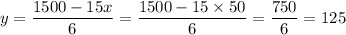
So, the largest dimensions that are possible would be 50 foot by 125 foot.