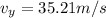Question

A cannonball is catapulted toward a castle. The cannonball’s velocity when it leaves the catapult is 51.6 m/s at an angle of 37.0° with respect to the horizontal, and the cannonball is 7.00 m above the ground at this time. The y-axis points up. What is the y-component of the cannonball’s velocity just before it lands?

Answer 1

`v_(y)=35.21m/s`

Step-by-step explanation:

From the exercise we know the cannonball's initial velocity, the angle which its released with respect to the horizontal and its initial height

`v_(o)=51.6m/s\\\beta =37.0º\\y_(o)=7m`

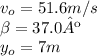
If we want to know whats the y-component of velocity we need to use the following formula:

`v_(y)^2=v_(oy)^2+ag(y-y_(o))`

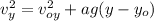
Knowing that
`g=-9.8m/s^2`

`v_(y)=√(((51.6m/s)sin(37))^2-2(9.8m/s^2)(0m-7m))=35.21m/s`

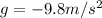
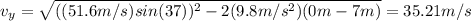
So, the cannonball's y-component of velocity is
`v_(y)=35.21m/s`