Question

A golf ball is given an initial velocity of 100 m/s at an angle of 22o with the horizontal. The golf ball lands on the roof of a building 50 m high.

a. Compute the initial vx and vy

b. Find the time it takes the ball to reach its maximum height

c. Find the velocity of the ball at its maximum height.

d. Find the maximum height of the golf ball.

e. Find the time the golf ball is in the air

Answer 1

a) 92.7 m/s, 37.5 m/s

The initial horizontal and vertical velocity are given by the equations:

`u_x = u cos \theta\\u_y = u sin \theta`

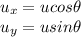
where

u = 100 m/s is the initial velocity of the ball

`\theta=22^(\circ)` is the angle of projection

So, the horizontal component of the initial velocity is

`u_x = (100)(cos 22)=92.7 m/s`

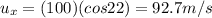
While the vertical component is

`u_y = (100)(sin 22)=37.5 m/s`

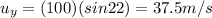
b) 3.83 s

The time at which the ball reaches the maximum height can be found by analyzing the vertical motion only.

In fact, the vertical velocity of the ball is given by

`v_y = u_y +at`

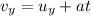
where

`u_y = 37.5 m/s`

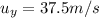
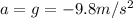`a=g=-9.8 m/s^2` is the acceleration of gravity (in the downward direction)

t is the time

The ball reaches the maximum height when the vertical velocity becomes zero:

`v_y = 0`

So, solving for t, we find the time at which this happens:

`t=-(u_y)/(g)=-(37.5)/(9.8)=3.83 s`

c) 92.7 m/s

As stated in part b), the vertical velocity of the ball at the maximum height is zero:

`v_y = 0`

Along the horizontal direction, insteand, the motion of the ball is uniform, since there are no forces acting in this direction. Therefore, the horizontal velocity of the ball is constant (because the horizontal acceleration is zero), and it is

`v_x = u_x = 92.7 m/s`

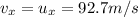
And therefore, the velocity of the ball at the maximum height is simply equal to the horizontal velocity:

`v=92.7 m/s`

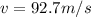
d) 71.7 m

The maximum height of the ball can be found by studying again the vertical motion only, by using the following suvat equation:

`s=u_yt+(1)/(2)at^2`

where

s is the vertical displacement at time t

`u_y = 37.5 m/s`

`a=g=-9.8 m/s^2`

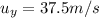
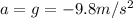
We know that the ball reaches the maximum height when t = 3.83 s, so we can find the vertical displacement at that time:

`s=(37.5)(3.83)+(1)/(2)(-9.8)(3.83)^2=71.7 m`

So, the maximum heigth is 71.7 m.

e) 5.93 s

We know that the ball lands on a building whose height is

h = 50 m

This means that when the ball lands, its vertical displacement is

s = 50 m

So, we can use again the equation

`s=u_yt+(1)/(2)at^2`

To find t, the time at which the ball lands. Substituting:

`u_y = 37.5 m/s`

`a=g=-9.8 m/s^2`

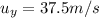
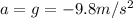
s = 50 m

The equation becomes

`50=37.5 t -4.9 t^2\\4.9t^2 -37.5 t +50 = 0`

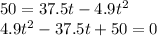
which gives two solutions:

t = 1.72 s

t = 5.93 s

The first solution is the moment at which the ball reaches the height of h = 50 m for the first time (before reaching its maximum height), so we ignore it, and therefore the ball lands after

t = 5.93 s