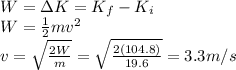(a) 638.4 J
The work done by a force is given by
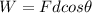
where
F is the magnitude of the force
d is the displacement of the object
 is the angle between the direction of the force and the displacement
is the angle between the direction of the force and the displacement
Here we want to calculate the work done by the force F, of magnitude
F = 152 N
The displacement of the suitcase is
d = 4.20 m along the ramp
And the force is parallel to the displacement, so
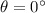 . Therefore, the work done by this force is
. Therefore, the work done by this force is
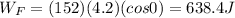
b) -328.2 J
The magnitude of the gravitational force is
W = mg
where
m = 19.6 kg is the mass of the suitcase
 is the acceleration of gravity
is the acceleration of gravity
Substituting,
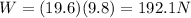
Again, the displacement is
d = 4.20 m
The gravitational force acts vertically downward, so the angle between the displacement and the force is
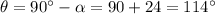
Where
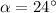 is the angle between the incline and the horizontal.
is the angle between the incline and the horizontal.
Therefore, the work done by gravity is
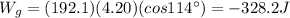
c) 0
The magnitude of the normal force is equal to the component of the weight perpendicular to the ramp, therefore:
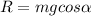
And substituting
m = 19.6 kg
g = 9.8 m/s^2

We find

Now: the angle between the direction of the normal force and the displacement of the suitcase is 90 degrees:
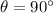
Therefore, the work done by the normal force is
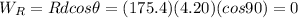
d) -194.5 J
The magnitude of the force of friction is
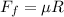
where
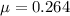 is the coefficient of kinetic friction
is the coefficient of kinetic friction
R = 175.5 N is the normal force
Substituting,

The displacement is still
d = 4.20 m
And the friction force points down along the slope, so the angle between the friction and the displacement is
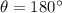
Therefore, the work done by friction is
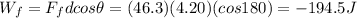
e) 115.7 J
The total work done on the suitcase is simply equal to the sum of the work done by each force,therefore:
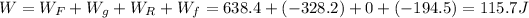
f) 3.3 m/s
First of all, we have to find the work done by each force on the suitcase while it has travelled a distance of
d = 3.80 m
Using the same procedure as in part a-d, we find:
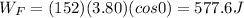
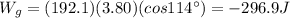
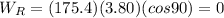
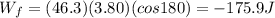
So the total work done is
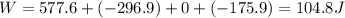
Now we can use the work-energy theorem to find the final speed of the suitcase: in fact, the total work done is equal to the gain in kinetic energy of the suitcase, therefore