Question

In the 18th century, the Chevalier de Mere asked Blaise Pascal to compare the probabilities of two events. Below, you will compute the probability of the two events that, prior to contrary gambling experience, were thought by de Mere to be equally likely. a b What is the probability of obtaining at least one 6 in four rolls of a fair die? If a pair of fair dice is tossed 24 times, what is the probability of at least one double six?

Answer 1

The probability of obtaining at least one 6 in four rolls of a fair die is 0.5177

The probability of obtaining at least one double six in 24 rolls of a fair die is 0.4914

Explanation:

Consider the provided information.

Part (A) What is the probability of obtaining at least one 6 in four rolls of a fair die?

The probability of getting a six in a throw of a fair die is = 1/6

The probability of not getting a six is = 5/6

Let X be the favorable outcome, that at least one 6.

To find the probability of at least one 6, subtract the probability that in 4 throws of a die no 6 is obtained from 1.

`1-((5)/(6))^4=0.5177`

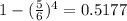
Hence, the probability of obtaining at least one 6 in four rolls of a fair die is 0.5177

Part (B) If a pair of fair dice is tossed 24 times, what is the probability of at least one double six?

Throwing two die at a time then the probability of two six is = 1/36

Throwing two die at a time then the probability of not getting two six is = 35/36

To find the probability of at least one double 6, subtract the probability that in 24 throws of a die no 6 is obtained from 1.

`1-((35)/(36))^(24)=0.4914`

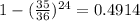
Hence, the probability of obtaining at least one double six in 24 rolls of a fair die is 0.4914