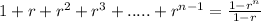Answer:
Explanation:
Taking the succession:

You can multiply and divide by 1-r without chaging the result:
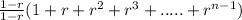
Distributing the upper part of the fraction you have:
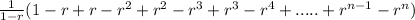
As can be seen all the intermediate members will be canceled by a same member with opposite sign, only
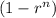 will be left so:
will be left so: