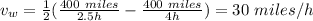Answer:
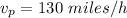
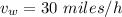
Explanation:
Lets call
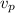 the the still-air speed of the plane and
the the still-air speed of the plane and
 the speed of the wind. On the first part the speed of the plane relative to the ground will be
the speed of the wind. On the first part the speed of the plane relative to the ground will be
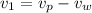 , and on the second part it will be
, and on the second part it will be
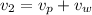 .
.
We know that the distance d=400 miles on the first and second parts are the same, so by the definition of velocity we will have:


Or:
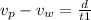
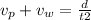
Adding both equations:
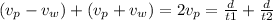
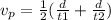
Which for our values is:
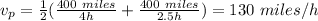
If instead of adding, we substact, we would have:
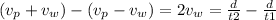
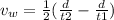
Which for our values is: