Question

Prove that if x > 1, then a triangle with side lengths x^2 − 1, 2x, and x^2+ 1 is a right triangle.

Answer 1

See the explanation.

Explanation:

If a triangle is a right triangle, then it should follow pythagoras theorem.

So sum of squares of two sides is equal to the square of the third longest side.

`(x^(2) -1 )^(2)   = x^(4) - 2 x^(2)  + 1`

`(x^(2) +1 )^(2)   = x^(4) + 2 x^(2)  + 1`

`(2x)^(2)  = 4x^(2)`

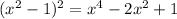
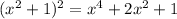
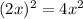
Adding the first and third equation we get the second equation.

So it follows Pythagoras theorem.

Hence it is a right triangle with
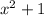`x^(2) + 1` as the hypotenuse.