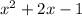Question

Rewrite each expression as a polynomial in standard form: x^2-5x+6 ÷ x-3 + x^3-1 ÷ x-1

Answer 1

So in standard form equation will be
`x^2+2x-1`

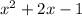
Explanation:

We have given expression
`(x^2-5x+6)/(x-3)+(x^3-1)/(x-1)`

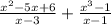
Let first we solve first part of the expression

So
`(x^2-5x+6)/(x-3)=(x^2-3x-2x+6)/(x-3)=((x-3)(x-2))/(x-3)=x-2`

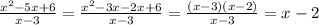
Now second part
`(x^3-1)/(x-1)`

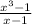
We know the algebraic identity
`a^3-b^3=(a-b)(a^2+ab+b^2)`

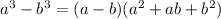
So by using this identity

`(x^3-1)/(x-1)=((x-1)(x^2+x+1))/(x-1)=x^2+x+1`

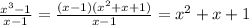
Now adding first and second part

`x-2+x^2+x+1=x^2+2x-1`

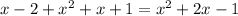
So in standard form equation will be
`x^2+2x-1`