Question

Compare the domains of the logarithmic function f(x) and the square root function g(x).

In two or more complete sentences, explain whether or not the domains of the two functions are the same.

Answer 1

The domains are not the same

Explanation:

The domains are not the same because in the first graph, the left part of the graph is always approaching 2, not reaching it, while the right side is extending infinitely. In the second graph, the left side DOES reach three except it doesn't cross it.

So the notation for the first line would be

2<x<infinity

2\leq x <infinity

Answer 2

Domains aren't the same.

Explanation:

These functions have different behaviours. First, the square root function doesn't admit negative values neither in y or x sets, because they are undetermined, but a logarithmic function admits negative values in one set. So, basically the graph of a squared root has place only in the first quadrant and the logarithmic function have place in two quadrants.

Also, each domain are like:

`D_(root) = (x\geq 0)\\D_(log) = (x > 0)\\`

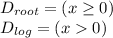
When we write each domain we can see that the main difference is the zero. A squared root function admits the zero, but a logarithmic function doesn't.

Therefore, they are not the same.