Answer:
Correct options are A and D.
Explanation:
From the given graph it is clear that the spaceship is located at (-4,3). The alien is at (-2, -3).
If a line passes through two points
 and
and
 , then the equation of line is
, then the equation of line is
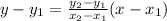
The equation of line is
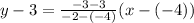
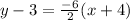

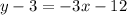
Add 3 on both sides.
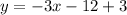
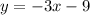 .... (1)
.... (1)
Slope intercept form of a line is
 .... (2)
.... (2)
where, m is slope and b is y-intercept.
On comparing (1) and (2) we get
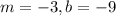
It means,
Slope = -3
y-intercept = -9
Points from -6 to 6 are labeled on y-axis. The y-intercept is -9, so it is not visible on the graph. Option A is correct.
Substitute y=0 in equation (1) to find the x-intercept.
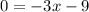

Divide both sides by 3.

x-intercept is -3. It means the line crosses the x-axis at -3. Option D is correct.