Question

Question 6 The mineral barite, (BaSO.) has a ke of 1.1 x 10" at 25°C. Calculate the solubility of barium sulfate in water, in: 6.1. moles per liter 6.2. grams per liter

Answer 1

Step-by-step explanation:

(6.1). The reaction equation will be as follows.

`BaSO_(4)(s) \rightleftharpoons Ba^(2+)(aq) + SO^(2-)_(4)(aq)`

Assuming the value of
`K_(sp)` as
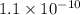`1.1 * 10^(-10)` and let the solubility of each specie involved in this reaction is "s". The expression for
`K_(sp)` will be as follows.

`K_(sp) = [Ba^(2+)][SO^(-)_(2)]` (Solids are nor considered)

=
`s * s`

s =
`\sqrt{K_(sp)}`

=
`\sqrt{1.1 * 10^(-10)}`

=
`1.05 * 10^(-5)`

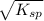
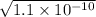
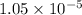
Therefore, solubility of barium sulfate in water is
`1.05 * 10^(-5)`.

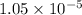(6.2). As the molar mass of
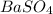`BaSO_(4)` is 233.38 g/mol

Therefore, the solubility is g/L will be calculated as follows.

`233.38 g/mol * 1.05 * 10^(-5)`

=
`2.45 * 10^(-3) g/L`

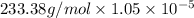
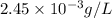
Therefore, solubility of barium sulfate in grams per liter is
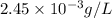`2.45 * 10^(-3) g/L`.