Question

A cone fits inside a square pyramid as shown. For every cross section, the ratio of the area of the circle to the area of the square is StartFraction pi r squared Over 4 r squared EndFraction or StartFraction pi Over 4 EndFraction.

A cone is inside of a pyramid with a square base. The cone has a height of h and a radius of r. The pyramid has a base length of 2 r.

Since the area of the circle is StartFraction pi Over 4 EndFraction the area of the square, the volume of the cone equals

A. StartFraction pi Over 4 EndFraction the volume of the pyramid or StartFraction pi Over 4 EndFractionStartFraction pi Over 4 EndFraction (StartFraction (2 r) (h) Over 3 EndFraction) or One-sixthπrh.

B. StartFraction pi Over 4 EndFraction the volume of the pyramid or StartFraction pi Over 4 EndFractionStartFraction pi Over 4 EndFraction (StartFraction (2 r) squared (h) Over 3 EndFraction) or One-thirdπr2h.

C. StartFraction pi Over 2 EndFraction the volume of the pyramid or StartFraction pi Over 2 EndFraction or Two-thirdsπr2h.

D. StartFraction pi Over 2 EndFraction the volume of the pyramid or StartFraction pi Over 4 EndFraction or One-thirdπr2h.

Answer 1

b

Explanation:

Answer 2

Option B

Explanation:

we know that

The volume of the cone is equal to

`V_c=(1)/(3)B_c(h)`

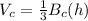
where

Bc is the area of the circle of the base of the cone

The volume of the square pyramid is equal to

`V_p=(1)/(3)B_p(h)`

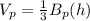
where

Bp is the area of the square base of the pyramid

we know that

`(B_c)/(B_p)=(\pi)/(4)`

`B_c=(\pi)/(4)(B_p)`

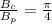
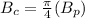
substitute in the formula of volume of the cone

`V_c=(1)/(3)B_c(h)`

`V_c=(1)/(3)((\pi)/(4)(B_p))(h)`

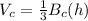
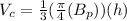
Remember that

`V_p=(1)/(3)B_p(h)`

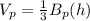
substitute

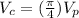`V_c=((\pi)/(4))V_p` ----> StartFraction pi Over 4 EndFraction the volume of the pyramid

or

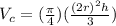`V_c=((\pi)/(4))(((2r)^2h)/(3))` ----> StartFraction pi Over 4 EndFraction (StartFraction (2 r) squared (h) Over 3 EndFraction)

or

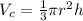`V_c=(1)/(3)\pi r^(2) h` ----> One-thirdπr^2h