Question

Problem 4. Prove that log, 11 is irrational (15 pts) Answer:

Answer 1

Answer and explanation:

To prove :
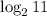`\log_2 11` is irrational ?

Proof :

Let
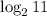`\log_2 11` is rational number.

So, It can be expressed in p/q form where, p and q are integers and q is non-zero.

`\log_2 11=(p)/(q)`

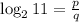
Using property of logarithm,

`11=(2)^{(p)/(q)}`

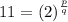
or
`11^q=(2)^(p)`

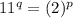
Which means 11 must be divisible by 2 for some p and q,

But 11 and 2 are co-prime.

So, Our assumption is not true.

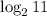`\log_2 11` is irrational number.

Hence proved.