Answer:
The distance between Eddie's home and the school is 3000 m
Explanation:
Consider the provided information.
His sister started walking at 8.00 am at an average speed of 50 m/min. Eddie started walking at 8.05 am at a speed of 60 m/min. Eddie caught up with his sister at mid-point between the school and his home.
Let the distance between school and home is x.
Let t is the time taken by Eddie sister to reach at mid-point between school and home.
Eddie caught up with his sister at mid-point between the school and his home.
That means his sister covers x/2 distance.
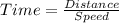
Speed of Eddie sister is 50 m/min.
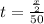 .....(1)
.....(1)
Eddie started walking at 8.05 am at a speed of 60 m/min.
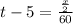
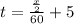 .....(1)
.....(1)
Equate both the equation as shown:
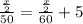
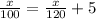
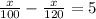
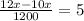
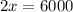
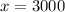
Hence, the distance between Eddie's home and the school is 3000 m