Question

An electron of mass 9.11 10-31 kg has an initial speed of 3.80 105 m/s. It travels in a straight line, and its speed increases to 7.60 105 m/s in a distance of 4.20 cm. Assume its acceleration is constant. (a) Determine the magnitude of the force exerted on the electron. 0.00000000000000000082875492 Incorrect: Your answer is incorrect. Your response differs from the correct answer by more than 10%. Double check your calculations. N (b) Compare this force (F) with the weight of the electron (Fg), which we ignored. F Fg =

Answer 1

`F=4.7*10^(-18)N`

`(F)/(W)=525702635794`

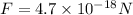
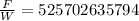
Step-by-step explanation:

The acceleration experimented by the electron can be calculated using the formula
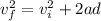`v_f^2=v_i^2+2ad`, so we have:

`a=(v_f^2-v_i^2)/(2d)`

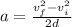
The magnitude of of the force exerted on the electron can be then obtained using Newton's 2nd Law F=ma.

Putting all together we have:

`F=ma=(m(v_f^2-v_i^2))/(2d)`

And using our values (and that 4.2cm are 0.042m):

`F=((9.11*10^(-31)Kg)((7.6*10^5m/s)^2-(3.8*10^5m/s)^2))/(2(0.042m))=4.7*10^(-18)N`

Then we compare this force with the weight of the electron:

`(F)/(W)=((m(v_f^2-v_i^2))/(2d))/(mg)=((v_f^2-v_i^2))/(2dg)=((7.6*10^5m/s)^2-(3.8*10^5m/s)^2)/(2(0.042m)(9.81m/s^2))=525702635794`

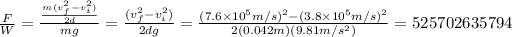
So this force is 525702635794 times larger than the weight of the electron.