Answer:
The magnitude of the force is 124.23 N.
Step-by-step explanation:
To known the magnitude of the force is necessary to find the acceleration, that can be done by means of the equations for a a Uniformly Accelerated Motion:
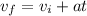 (1)
(1)
Where
 is the final velocity,
is the final velocity,
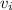 is the initial velocity, a is the acceleration and t is the time.
is the initial velocity, a is the acceleration and t is the time.
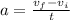 (2)
(2)
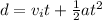 (3)
(3)
By replacing (2) in equation (3) it is gotten:
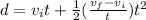


Therefore, by subtracting the first and third term (
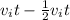 ⇒
⇒
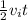 ) it is got:
) it is got:
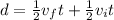
Applying common factor for
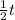 :
:
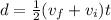 (4)
(4)
Equation (4) can be rewritten in terms of t:
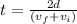



By knowing the time is possible to determine the acceleration by means of equation (2):
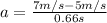
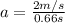
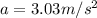
The magnitude of the force exerted by the person on the block can be determine by means of Newton's second law:
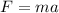 (5)
(5)
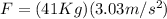
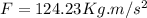

Hence, the magnitude of the force is 124.23 N.