Question

What is that standard deviation of the data set? round to the nearest tenth if needed.

2,4,7,8,9
​

Answer 1

Answer: Just took the test. 2.6 was marked incorrect and 2.9 was shown as the correct answer

Explanation:

Answer 2

Standard deviation of 2, 4, 7, 8, 9 is 2.6

SOLUTION:

Given, data set is 2, 4, 7, 8, 9.

We know that, Standard deviation is given by

`\sigma=\sqrt{(\Sigma(X i-\mu)^ 2)/(n)}`

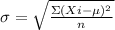
Where,
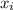`x_(i)` is element of data set

`\mu` is mean of data set

n is total number observations.

Now, mean is given by

`\mu=\frac{s u m o f \text { observations }}{\text {number of observations}}`

`\begin{array}{l}{=(2+4+7+8+9)/(5)} \\\\ {=(30)/(5)}\end{array}`

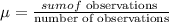
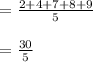
= 6

So, the mean of data set is 6.

Now, standard deviation,

`\sigma=\sqrt{((2-6)^2+(4-6)^2+(7-6)^2+(8-6)^2+(9-6)^2)/(5)}`

`\sigma=\sqrt{((-4)^2+(-2)^2+(1)^2+(2)^2+(3)^2)/(5)}`

`\begin{array}{l}{\sigma=\sqrt{(16+4+1+4+9)/(5)}} \\\\ {\sigma=\sqrt{(34)/(5)}} \\\\ {\sigma=√(6.8)}\end{array}`

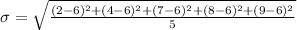
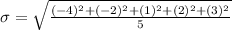
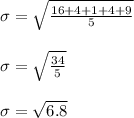
So, the standard deviation is 2.607 approximately.

When rounded to nearest tenth answer is 2.6