Question

A clinical trial was conducted to test the effectiveness of the drug zopiclone for treating insomnia in older subjects. Before treatment with zopiclone, 16 subjects had a mean wake time of 102.8 minutes. After treatment with zopiclone, the 16 subjects had a mean wake time of 98.9 minutes and s standard deviation of 42.3 minutes. Assume that the 16 sample values appear to be from a normally distributed population and construct a 98% confidence interval estimate of the mean wake time for a population with zopiclone treatments. What does the result suggest about the mean wake time of 102.8 minutes before the treatment? Does zopiclone appear to be effective?

Answer 1

We cannot say that the mean wake time are different before and after the treatment, with 98% certainty. So the zopiclone doesn't appear to be effective.

Explanation:

The goal of this analysis is to determine if the mean wake time before the treatment is statistically significant. The question informed us the mean wake time before and after the treatment, the number of subjects and the standard deviation of the sample after treatment. So using the formula, we can calculate the confidence interval as following:

`IC[\mu ; 98\%] = \overline{y} \pm t_(0.99,n-1)\sqrt{(Var(y))/(n)}`

Knowing that
`t_(0.99,15) = 2.602`:

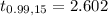`IC[\mu ; 98\%] = 98.9 \pm 2.602(42.3)/(4) \Rightarrow 98.9 \pm 27.516`

`IC[\mu ; 98\%] = [71.387 ; 126,416]`

Note that
`102.8 \in [71.384 ; 126.416]` so we cannot say, with 98% confidence, that the mean wake time before treatment is different than the mean wake time after treatment. So the zopiclone doesn't appear to be effective.