Question

Rachel hikes at a steady rate from a ranger station to a campground that is 20 mi away. After 2 h, she is 13 mi from the campground. After 4 h, she is 6 mi from the campground. A graph shows her distance from the campground Y, in miles, after x hours. What is the slope of the graph and what does it represent?

Answer 1

-3.5

The distance Rachel covers per hour is 3.5 miles

Explanation:

After 2 hours, she is 13 miles from the campground.

After 4 hours, she is 6 miles from the campground.

Let x be the number of hours, y be the number of miles from the campground, then we have two points (2,13) and (4,6).

The equation of the line passing through the points
`(x_1,y_1)` and
`(x_2,y_2)` is

`y-y_1=(y_2-y_1)/(x_2-x_1)(x-x_1)`

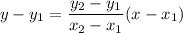
Substitute:

`x_1=2\\ \\y_1=13\\ \\x_2=4\\ \\y_2=6`

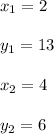
Hence,

`y-13=(6-13)/(4-2)(x-2)\\ \\y-13=-3.5(x-2)\\ \\y=-3.5x+7+13\\ \\y=-3.5x+20`

The slope of the line is
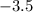`-3.5` and it represents that the distance Rachel covers per hour is 3.5 miles.