Answer:
The value of x in the given equation is 0.
Solution:
We know that any number with power 0 is 1
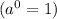
As given,
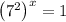
As per the formula,
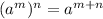
Here a is 7; 2 is m and x is n.
On substituting the values,
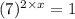
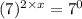 (//any power of 0 is 1)
(//any power of 0 is 1)
Hence from both the side we get,
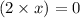
So, x = 0.