Question

Suppose that a company selects two people who work independently inspecting two-by-four timbers. Their job is to identify low-quality timbers. Suppose that the probability that an inspector does not identify a low-quality timber is 0.20. (a) What is the probability that both inspectors do not identify a low-quality timber? (b) How many inspectors should be hired to keep the probability of not identifying a low-quality timber below 1%? (c) Interpret the probability from part (a).

Answer 1

Explanation:

Given

Probability that an inspector doe not identify low-quality timber=0.20

(a)Probability that both inspector do not identify a low quality timber

`P=0.2* 0.2=0.04`

(b)Let n be the no of inspector

Probability should be less than 1%=0.01

so
`\left ( 0.2\right )^n<0.01`

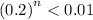
Taking
`\ln`both sides

`x>(\ln 0.01)/(\ln 0.2)`

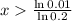
x>2.861

thus minimum value of x is 3

(c)From part b it is clear that minimum 3 officer are required to to keep the low quality timber below 1%

on an average 2 officer are not sufficient to identify 4 low quality timber out of 100