Question

Factor x3 + 2x2 + x completely.

a. (x+1)^2

b. x(x+1)^2

c. x(x+1)^2

Answer 1

factor x3+2x2+x

answer;D

Answer 2

Option B

ANSWER:

The factors of
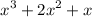`$x^(3)+2 x^(2)+x$` is
`$x(x+1)^(2)$`

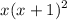
SOLUTION:

Given, cubic expression is
`$x^(3)+2 x^(2)+x$`

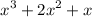
Now, we have to find the factors of above equation.

To factorize the given equation, follow the below steps:

`$\mathrm{x}^(3)+2 \mathrm{x}^(2)+\mathrm{x}$`

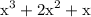
Since x is common in every term of expression, we can take it as common

`$x\left(x^(2)+2 x+1\right)$`

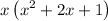
“2x” can be rewritten as “x + x”, the above equation becomes,

`$x\left(x^(2)+x+x+1\right)$`

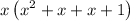
Taking the common terms out of bracket. we get

x(x (x + 1) + 1 (x + 1))

Taking (x + 1) as common., we get

x ((x + 1)(x + 1))

`$x(x+1)^(2)$`

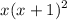
Hence, the second option b is correct.