Answer:
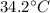
Step-by-step explanation:
The resistance increases linearly with the temperature - so we can write:

where
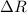 is the change in resistance
is the change in resistance
k is the coefficient of proportionality
 is the variation of temperature
is the variation of temperature
In the first part of the problem, we have
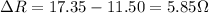
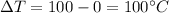
So the coefficient of proportionality is
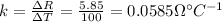
When the resistance is
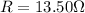 , the change in resistance with respect to the resistance at zero degrees is
, the change in resistance with respect to the resistance at zero degrees is
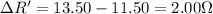
So we can find the change in temperature as:

So the new temperature is
