Question

Surface Area of a can is 517.8 cm^2. Maximize the volume of this can using the measured surface area.

Answer 1

`r = 5.24` --- Radius

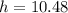`h = 10.48` --- Height

Step-by-step explanation:

Given

Object: Can (Cylinder)

`Surface\ Area = 517.8cm^2`

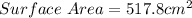
Required

Maximize the volume

The surface area is:

`S.A = 2\pi r^2 + 2\pi rh`

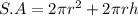
Substitute 517.8 for S.A

`517.8 = 2\pi r^2 + 2\pi rh`

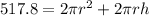
Divide through by 2

`258.9 = \pi r^2 + \pi rh`

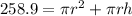
Factorize:

`258.9 = \pi r(r + h)`

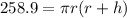
Divide through by
`\pi r`

`(258.9)/(\pi r) = r + h`

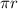
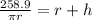
Make h the subject

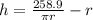`h = (258.9)/(\pi r) - r` --- (1)

Volume (V) is calculated as:

`V = \pi r^2h`

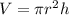
Substitute (1) for h

`V = \pi r^2((258.9)/(\pi r) - r)`

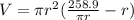
Open Bracket

`V = 258.9r - \pi r^3`

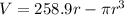
Differentiate V

`V' = 258.9 - 3\pi r^2`

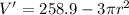
Set V' to 0

`0 = 258.9 - 3\pi r^2`

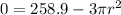
Collect Like Terms

`3\pi r^2 = 258.9`

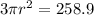
Divide through by 3

`\pi r^2 = 86.3`

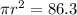
Divide through by
`\pi`

`r^2 = (86.3)/(\pi)`

`r^2 = (86.3*7)/(22)`

`r^2 = (604.1)/(22)`

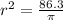
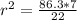
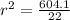
Take square root of both sides

`r = \sqrt{(604.1)/(22)`

`r = 5.24`

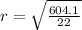
Recall that:

`h = (258.9)/(\pi r) - r`

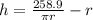
Substitute 5.24 for r

`h = (258.9)/(\pi * 5.24) - 5.24`

`h = (258.9*7)/(22 * 5.24) - 5.24`

`h = (1812.3)/(115.28) - 5.24`

`h = 15.72 - 5.24`

`h = 10.48`

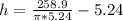
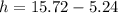
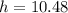
Hence, the dimension that maximize the volume is:

`r = 5.24` --- Radius

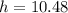`h = 10.48` --- Height