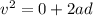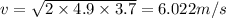Question

You are designing an elevator for a hospital. The force exerted on a passenger by the floor of the elevator is not to exceed 1.59 times the passenger's weight. The elevator accelerates upward with constant acceleration for a distance of 3.7 m and then starts to slow down. What is the maximum speed of the elevator?

Answer 1

The maximum speed is 6.022 m/s

Solution:

As per the question:

Distance covered by the elevator in the upward direction, d = 3.7 m

Maximum force exerted by the elevator on the passenger = 1.59 w N

where

w = weight of the passenger = mg

where

m = mass of the passenger

g = acceleration due to gravity =
`9.8 m/s^(2)`

Therefore,

Maximum force exerted by the elevator on the passenger = 1.59mg N

Now, for the maximum speed of the elevator:

Net force on the floor of the elevator when it moves upwards:

`F_(net) = m(g + a)`

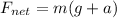
Thus

For maximum acceleration:

`1.5 mg = m(g + a)`

`1.5 g = g + a`

`a = 0.5 g = 0.5* 9.8 = 4.9 m/s^(2)`

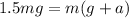
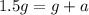
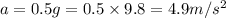
Now, from the third eqn of motion with initial velocity 0:

`v^(2) = 0 + 2ad`

`v = √(2* 4.9* 3.7) = 6.022 m/s`

Answer 2

maximum speed of the elevator is 6.54 m/s

Step-by-step explanation:

given,

force exerted on the elevator is 1.59 times passenger weight.

when the elevator accelerates upward with rate of a.

total force

F = m × (a+g)

1.59× mg = ma + mg

0.59 mg = ma

a = 0.59 g

we also have that

`v^2 = u^2+ 2 a s`

`v =√(2a*s)`

=
`\sqrt(2* 0.59* 9.8* 3.7)`

= 6.54 m/s

maximum speed of the elevator is 6.54 m/s