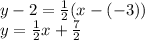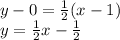Question

Find equations of the tangent lines to the curve y = ( x − 1) / (x + 1) that are parallel to the line x − 2y = 5.

Answer 1

`y = (1)/(2)x+(7)/(2)`

`y = (1)/(2)x-(1)/(2)`

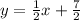
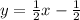
Explanation:

The first step is obtain the derivative of the function y:

`(dy)/(dx)=((x+1)*(1)-(x-1)*1)/((x+1)^(2) )=(2)/((x+1)^(2) )`

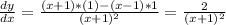
(Remember that the slope of the tangent line to any curve is given by its derivative).

Secondly, in order to obtain the equations of the tangent lines parallel to x - 2y = 5 we need to obtain the slope of this line, remember that the line equation is given by:

`y = mx + b`

m : slope

b: y-intercept

Then, the slope of the line

`y = (1)/(2)x-(5)/(2)`

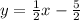
is m = 1/2.

Then, the derivative of the function y must be 1/2:

`y= (2)/((x+1)^(2))=(1)/(2)\\(x+1)^(2)=4\\x^(2)+2x-3=0\\ (x+3)(x-1)=0\\x = -3\\x=1`

When x = -3, y =(-3-1)/(-3+1)= 2, then one of the tangent lines must pass through the point (-3,2).

When x = 1, y = (1-1)(1+1)= 0, then the other tangent line must pass through the point (1, 0).

Finally, the point-slope equation of a line is given by:

`y- y_(1)  = m(x - x_(1))`

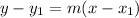
Substituting our previous results we have the equations of the tangent lines:

`y - 2 = (1)/(2)(x - (-3))\\ y = (1)/(2)x+(7)/(2)`

`y - 0 = (1)/(2)(x- 1) \\y = (1)/(2)x-(1)/(2)`