Question

In nuclear fission and fusion reactions mass is converted into energy. The average person in US uses around 100,000,000 J of energy per year.

(a) Calculate the yearly energy consumption for a US population of 300 million.
(b) Using Einstein's famous formula, E = m c^2, calculate the energy that would be released if a 60 kg person were converted entirely into energy.
(c) How many years would this amount of energy support a population of 300 million?

Answer 1

Step-by-step explanation:

2/7 = 8/28

1/4 = 7/28

Therefore Mr Pham mowed more.

8+7 = 15 so 15/28 of lawn has been mowed, therefore 13/28 of the lawn is left still to be mowed. NO RIGHT

Answer 2

a) The yearly energy consumption for a US population of 300 million is
`3* 10^(16) J/year`

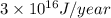
b) The energy that would be released if a 60 kg person were converted entirely into energy is
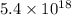`5.4* 10^(18)` Joules.

c) 180 years would this amount of energy support a population of 300 million.

Step-by-step explanation:

a) Average energy consumed by single person of US = 100,000,000 J/year

Then 300 million US citizen will consume:

300 million = 300 × 1,000,000 =
`3* 10^8`

The yearly energy consumption for a US population :

`100,000,000 J/year* 3* 10^8=3* 10^(16) J/year`

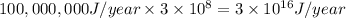
The yearly energy consumption for a US population of 300 million is
`3* 10^(16) J/year`

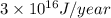
b)
`E = m* c^2`

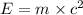
E = Energy from converted mass of m

c = speed of light

Given mass of person = m = 60 kg

`E=60 kg* (3* 10^8 m/s)^2 = 5.4* 10^(18) J`

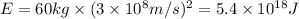
c) Energy calculated in part (b) =
`E=5.4* 10^(18) J`

The yearly energy consumption for a US population of 300 million in an year =
`3* 10^(16) J/year`

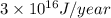
Let the that would be supported by
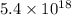`5.4* 10^(18)` Joules of energy be x.

`x* 3* 10^(16) J/year=5.4* 10^(18) J`

`x=(5.4* 10^(18) J)/(3* 10^(16) J/year)=180 years`

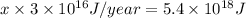
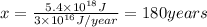
180 years would this amount of energy support a population of 300 million.