Answer:
The probability that X will be more than 0.5 away fro the population
mean is 0.7812 ⇒ answer a
Explanation:
* Let us explain how to solve the problem
- The sample size n is 25
- The mean of the population μ is 41
- The standard deviation σ is 9
- We need to find the approximate probability that X will be more than
0.5 away from the population mean
∵ z-score = (X - μ)/(σ/√n)
∵ X - μ = 0.5
- That means X > 41 + 0.5, OR X < 41 - 0.5
- Then we need to find P(X > 41.5) and P(X < 40.5)
∵ z =
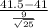 = 0.2778
= 0.2778
∵ z =
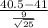 = -0.2778
= -0.2778
- Let us use the normal distribution table to find the corresponding
area to z-score
∵ P(z < 0.2778) = 0.6116
∵ P(z > -0.2778) = 0.3928
- P(40.5 < X < 41.5) = P( -0.2778 < z < 0.2778)
∴ The probability of X to be with in 0.5 = 0.6116 - 0.3928
∴ The probability of X to be with in 0.5 = 0.2188
∵ The probability of X to be more than 0.5 away from the population
mean = 1 - 0.2188
∴ P(X > 41.5) OR P(X < 40.5) = 0.7812
* The probability that X will be more than 0.5 away fro the population
mean is 0.7812