Answer:
(40.5;0) cm
Explanation:
We know that:
The length of the leg is:

The problem says that each part of the leg has the same length, that is:
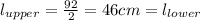
Also, the leg is uniform, so the center of mass of each part is in the middle, this is the position (r):
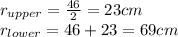
This means that the center of mass of the upper leg is 23cm from the hip, and the lower leg if 69c m from the hip.
In addition, we know each mass:

Now, we have all values needed, we use the proper equation to calculate the center of mass of the leg:
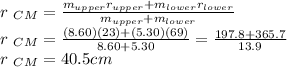
Therefore, the center of mass of the leg is 40.5m from the hip in a horizontal direction because it's stretched out horizontally Specifically, the coordinates are (40.5;0) cm.