Question

Bags of whole coffee beans are filled automatically on a production line. A machine fills each bag so that the weight of coffee beans inside is normally distributed with a mean of 290 grams. The label on the bag, however, states that the weight of coffee beans inside is 283 grams.What is the standard deviation of bags of coffee beans, if 13% of the bags have a weight below what is stated on the label?

Answer 1

Answer: The standard deviation would be 6.1947.

Explanation:

Since we have given that

Mean = μ = 290

Weight of coffee beans = x = 283 grams

Since P(Z<z)=13% = 0.13

We need to find the standard deviation:

Using the standard normal table,

P(Z<-1.13)=0.13

So, we get that

z = -1.13

Using the z-score formula, we get that

`x=z* \sigma+\mu\\\\283=-1.13* \sigma+290\\\\283-290=-1.13* \sigma\\\\-7=-1.13* \sigma\\\\(7)/(1.13)=\sigma\\\\\sigma=6.1947`

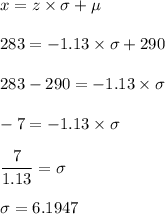
Hence, the standard deviation would be 6.1947.