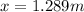Question

Spitting cobras can defend themselves by squeezing muscles around their venom glands to squirt venom at an attacker. Suppose a spitting cobra rears up to a height of 0.440 m above the ground and launches venom at 3.10 m/s, directed 47.0° above the horizon. Neglecting air resistance, find the horizontal distance (in m) traveled by the venom before it hits the ground.

Answer 1

Answer: 1.289 m

Step-by-step explanation:

The path the cobra's venom follows since it is spitted until it hits the ground, is described by a parabola. Hence, the equations for parabolic motion (which has two components) can be applied to solve this problem:

x-component:

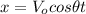`x=V_(o)cos\theta t` (1)

Where:

`x` is the horizontal distance traveled by the venom

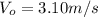`V_(o)=3.10 m/s` is the venom's initial speed

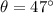`\theta=47\°` is the angle

`t` is the time since the venom is spitted until it hits the ground

y-component:

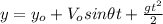`y=y_(o)+V_(o)sin\theta t+(gt^(2))/(2)` (2)

Where:

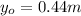`y_(o)=0.44 m` is the initial height of the venom

`y=0` is the final height of the venom (when it finally hits the ground)

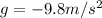`g=-9.8m/s^(2)` is the acceleration due gravity

Let's begin with (2) to find the time it takes the complete path:

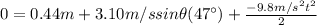`0=0.44 m+3.10 m/s sin\theta(47\°)+(-9.8m/s^(2) t^(2))/(2)` (3)

Rewritting (3):

`-4.9 m/s^(2) t^(2) + 2.267 m/s t + 0.44 m=0` (4)

This is a quadratic equation (also called equation of the second degree) of the form
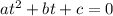`at^(2)+bt+c=0`, which can be solved with the following formula:

`t=\frac{-b \pm \sqrt{b^(2)-4ac}}{2a}` (5)

Where:

`a=-4.9 m/s^{2`

`b=2.267 m/s`

`c=0.44 m`

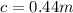
Substituting the known values:

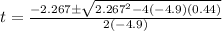`t=\frac{-2.267 \pm \sqrt{2.267^(2)-4(-4.9)(0.44)}}{2(-4.9)}` (6)

Solving (6) we find the positive result is:

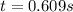`t=0.609 s` (7)

Substituting (7) in (1):

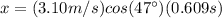`x=(3.10 m/s)cos(47\°)(0.609 s)` (8)

We finally find the horizontal distance traveled by the venom:

`x=1.289 m`