Question

A hunter wishes to cross a river that is 1.74 km wide and flows with a speed of 2.68 km/h parallel to its banks. The hunter uses a small powerboat that moves at a maximum speed of 16.9 km/h with respect to the water. What is the minimum time necessary for crossing? Answer in units of min.

Answer 1

7.32 mins

Step-by-step explanation:

Data:

Let the length of the river be = 1.74 km.

The speed of water = 2.68 km/h

The maximum speed of the boat = 16.9 km

Therefore, the time will be:

The speed of the boat relative to water = 16.9 - 2.68

= 14. 22 km/h

the time = 1.74/ 14.22

= 0.122 h

= 7.32 mins

Answer 2

Minimum time = 6.177 min

Step-by-step explanation:

We assume a reference system with the positive x-axis (from left to right) and also a positive y-axis (from bottom to top)

According to this system the velocity vector of the river and the hunter are :

`V_(hunter/river)=16.9 (km)/(h)i`

`V_(river/ground)=-2.68(km)/(h)j`

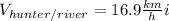
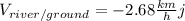
The velocity vector of the hunter relative to the ground is the sum of the previously mentioned velocities

`V_(hunter/ground)=16.9(km)/(h)i-2.68(km)/(h)j`

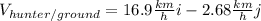
This means that,for example,in an hour the hunter moves 16.9 km in the positive x direction and 2.68 km in the negative y direction

We are looking for a displacement of 1.74 km in the x direction ⇒ We will use only the ''i'' component of the velocity

`speed=(distance)/(time) \\time=(distance)/(speed) \\time=(1.74km)/(16.9(km)/(h)) \\time = 0.102 h\\time = 6.177 min`

We multiply the time in hours by 60 to obtain the time in minutes

time T = 6.177 min