Question

Use the given zero to find the remaining zeros of each function f(x)=2x^4+5x^3+5x^2+20x-12 zero:-2i

Answer 1

The roots are -2i, 2i, 1/2 and -3.

Explanation:

The complex roots exist as conjugate pairs so if one root is -2i the other is 2i. So a factor of f(x) is (x - 2i)(2 + 2i). = x^2 + 4.

So dividing:

2x^2 + 5x - 3 <---- Quotient.

--------------------------------------------

x^2 + 0x + 4 ) 2x^4 + 5x^3 + 5x^2 + 20x - 12

2x^4 + 0x^3 + 8x^2

5x^3 - 3x^2 + 20x

5x^3 + 0x^2 + 20x

-3x^2 - 12

-3x^2 - 12.

Finding the other 2 zeroes:

2x^2 + 5x - 3 = 0

(2x - 1) ( x + 3) = 0

x = 1/2, -3.

Answer 2

1/2, 3

Explanation:

This is a pretty involved problem, so I'm going to start by laying out two facts that our going to help us get there.

1. The Fundamental Theorem of Algebra tells us that any polynomial has as many zeroes as its degree. Our function f(x) has a degree of 4, so we'll have 4 zeroes. Also,
2. Complex zeroes come in pairs. Specifically, they come in conjugate pairs. If -2i is a zero, 2i must be a zero, too. The "why" is beyond the scope of this response, but this result is called the "complex conjugate root theorem".

In 2., I mentioned that both -2i and 2i must be zeroes of f(x). This means that both
`x-2i` and
`x+2i` are factors of f(x), and furthermore, their product,
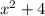`x^2+4`, is also a factor. To see what's left after we factor out that product, we can use polynomial long division to find that

`2x^4+5x^3+5x^2+20x-12=(x^2+4)(2x^2+5x-3)`

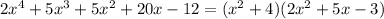
I'll go through to steps to factor that second expression below:

`2x^2+5x-3=2x^2+6x-x-3\\=2x(x+3)-(x+3)\\=(2x-1)(x+3)`

Solving both of the expressions when f(x) = 0 gets us our final two zeroes:

`2x-1=0\\2x=1\\x=1/2`

`x+3=0\\x=-3`

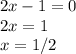
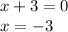
So, the remaining zeroes are 1/2 and 3.