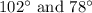Answer:
The measure of two supplementary angles are
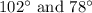
Solution:
Given that the difference of measure of the two angles is 24
Let the first angle be x and the second angle be x-24. We know when two angles are supplementary their sum is equal to
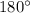
Now since x and (x-24) are two supplementary angles, therefore their sum will be equal to
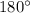
x+(x-24) = 180
2x-24=180
2x= 180+24
2x = 204
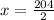
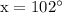
The first angle is
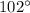
The second angle is (x – 24) so we get (102 - 24) = 78
Hence the measure of two supplementary angles are