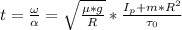Answer:
It will start to slip at
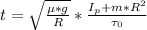
Step-by-step explanation:
We can find the angular acceleration from a sum of torque on the system:
 Solving for α
Solving for α

Now, on the object, we make a sum of forces on the centripetal-axis:
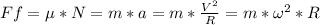 Solving for ω:
Solving for ω:
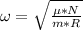
From a sum of forces on the axis perpendicular to the platform:
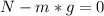
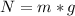
Replacing this value into the ω equation:
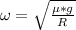 Now we have to find the amount of time that takes to the object to get this speed.
Now we have to find the amount of time that takes to the object to get this speed.
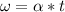 Solving for t:
Solving for t: