Step-by-step explanation:
As it is given that the initial concentration of sulfuric acid is 0.010 M.
Hence, the ICE table for first dissociation of sulfuric acid is as follows.

Initial: 0.010 0 0
Change: -0.010 +0.010 +0.010
Equilibrium: 0 0.010 0.010
When second dissociation of sulfuric acid occurs which is partial then the ICE table will be as follows.
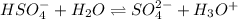
Initial: 0.010 0 0.010
Change: -x +x +x
Equilibrium: 0.010 - x x 0.010 + x
Since, it is given that
 . Hence, formula for
. Hence, formula for
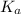 is as follows.
is as follows.
![K_(a) = ([SO^(2-)_(4)][H_(3)O^(+)])/([HSO^(-)_(4)])](https://img.qammunity.org/2020/formulas/chemistry/college/lhqrepqnpd89tjm3gdqkmczd4bgnv4k8r8.png)
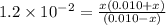
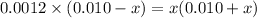
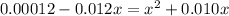
x = 0.0045
Hence, by using the equilibrium concentrations from the table and value of x we get the following.
-
![[SO^(2-)_(4)]](https://img.qammunity.org/2020/formulas/chemistry/college/a3lwwlgtn6ccxpmxiv2y31aykva4u82xbj.png) = x,
= x,
= 0.0045 M
-
![[HSO^(-)_(4)]](https://img.qammunity.org/2020/formulas/chemistry/college/ntsh7x8lij0v1m8uaru8od3hhjf3kjwiym.png) = 0.010 - x
= 0.010 - x
= 0.010 - 0.0045
= 0.0055 M
-
![[H_(3)O^(+)]](https://img.qammunity.org/2020/formulas/chemistry/high-school/9q4wjqeu25nf3f930xkrlsn4yiv6nzirb8.png) = 0.010 + x
= 0.010 + x
= 0.010 + 0.0045
= 0.0145 M