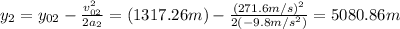Answer:
5080.86m
Step-by-step explanation:
We will divide the problem in parts 1 and 2, and write the equation of accelerated motion with those numbers, taking the upwards direction as positive. For the first part, we have:
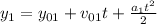
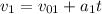
We must consider that it's launched from the ground (
 ) and from rest (
) and from rest (
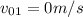 ), with an upwards acceleration
), with an upwards acceleration
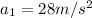 that lasts a time t=9.7s.
that lasts a time t=9.7s.
We calculate then the height achieved in part 1:
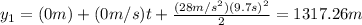
And the velocity achieved in part 1:
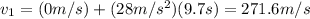
We do the same for part 2, but now we must consider that the initial height is the one achieved in part 1 (
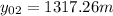 ) and its initial velocity is the one achieved in part 1 (
) and its initial velocity is the one achieved in part 1 (
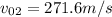 ), now in free fall, which means with a downwards acceleration
), now in free fall, which means with a downwards acceleration
 . For the data we have it's faster to use the formula
. For the data we have it's faster to use the formula
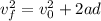 , where d will be the displacement, or difference between maximum height and starting height of part 2, and the final velocity at maximum height we know must be 0m/s, so we have:
, where d will be the displacement, or difference between maximum height and starting height of part 2, and the final velocity at maximum height we know must be 0m/s, so we have:
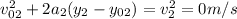
Then, to get
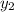 , we do:
, we do:
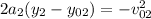

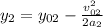
And we substitute the values: