Question

The volume of air in a certain climber​'s lungs​ (in cubic​ centimeters) is modeled by the​ equation: Upper V equals 350 sin (52 pi t )plus 800 where t is the time in minutes. Find the maximum volume of air in the climber​'s ​lungs, and determine how many breaths the climber takes per minute.

Answer 1

a) The maximun volume of air in the climber​'s ​lungs is
`1150 cm^(3)`

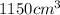
b) The climber takes 26 breaths per minute

Explanation:

a) The function is given by
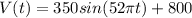`V(t)=350sin(52\pi t)+800`, where
`V(t)` is in
`cm^(3)`, and
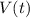`t` is in minutes.

So the first step is to derive it, by the chain rule we obtain
`V'(t)=(52\pi)350 cos(52 \pi t)`.

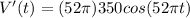After that, we make the expression equals to zero,
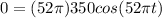`0=(52\pi)350 cos(52 \pi t)`, then
`0=cos(52 \pi t)`. This leads to
`cos^(-1)(0)=52 \pi t`.

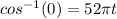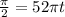`(\pi)/(2) =52 \pi t`, and clearing it for
`t=(\pi)/(2*52\pi)=(1)/(104)`.

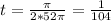The second step is to evaluate the original expression for this value so,
`V((1)/(104))=350sin(52\pi ((1)/(104)))+800=350sin ((\pi)/(2))+800=350+800=1150cm^(3)`.

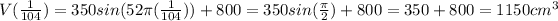b) As we can see this function have the form
`A*sin(\omega t)`, where
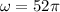`\omega=52\pi` is the angular frequency, so every
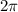`2 \pi` radians we will have a breath, therefore Breaths Per Minute=
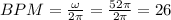`BPM=(\omega)/(2\pi)=(52\pi)/(2 \pi) =26`.