Answer:
0.0396
Explanation:
the probability of one page having an error is p= 2/100 = 1/50
if the letter q is the probability of not having an error then q = 49/50
Using binomial probability:
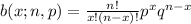
n is the sample size--> n = 20
And we want the probability that a random sample of 20 pages will contain at least one error, this is the same as 1 minus the probability of none of the 20 pages containing an error:
probability(x ≥ 1) = 1 - probability( x = 0)
Using the binomial probability equation
Probability( x=0 ) =
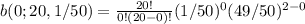
Probability( x=0 ) =
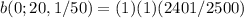 = 0.9604
= 0.9604
Thus,
probability(x ≥ 1) = 1 - 0.9604= 0.0396