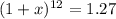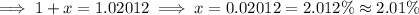Answer:
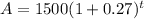
Rate of change per month would be 2.01%
Explanation:
Since, the exponential growth function,

Where,
P = principal amount,
r = annual rate,
n = number of compounding periods,
t = number of years,
Here,
P = 1500, r = 27% = 0.27, n = 1,
Thus, the number of users after t years,
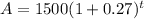
Let it is equivalent to number of users when it is growing at the rate of x monthly,
That is,
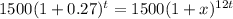
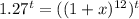
By comparing,