Question

An insured's roof cost $4,000 when installed 5 years ago. It has been damaged by hail and must be replaced. The new roof will cost $6,000 at today’s prices. If the roof has been depreciating at $200 per year and the insured’s policy is written on the actual cash value(ACV), how much will the policy pay toward the insured's new roof?

Answer 1

ACV=$4,500

Explanation:

We have that the actual cash value (ACV) is defined as:

`ACV=(R*(E-C))/(E)`

Where:

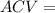`ACV =` actual cash value

`R =` replacement cost or purchase price of the item

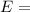`E =` expected life of the item

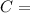`C =` current life of the item

Then we have R=$6,000, C=5years, and to find the expected life of the item we can use the depreciating of the roof, then if the roof is depreciating $200 each year we just need to divide $4,000 by $200 to find the expected life of the roof:

`(4,000)/(200)=20`

Then the espected life of the roof is 20 years, with this result we have all the data, then:

`ACV=(\$6,000* (20-5))/(20)=(\$6,000* (15))/(20)=(\$90,000)/(20)=\$4,500`

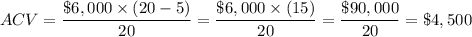
Then the ACV is $4,500