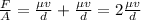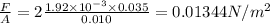Answer:
Force per unit plate area is 0.1344
Solution:
As per the question:
The spacing between each wall and the plate, d = 10 mm = 0.01 m
Absolute viscosity of the liquid,
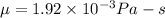
Speed, v = 35 mm/s = 0.035 m/s
Now,
Suppose the drag force that exist between each wall and plate is F and F' respectively:
Net Drag Force = F' + F''
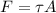
where
 = shear stress
= shear stress
A = Cross - sectional Area
Therefore,
Net Drag Force, F =
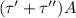

Also
F =
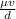
where
 = dynamic coefficient of viscosity
= dynamic coefficient of viscosity
Pressure, P =
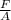
Therefore,