Answer:
V = 308.06 miles/hour
θ = 13.13° west of north.
Step-by-step explanation:
Airplane speed = V₁ = 300 miles/hour
Wind speed = V₂ = 70 miles/hour
Resultant speed of the plane = V = ?
As airplane is trying to fly straight North and wind is blowing toward the west. So the angle between airplane velocity and wind velocity is 90°.
By Pythagoras Theorem
V =
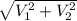
V =
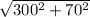
V = 308.06 miles/hour
θ = tan⁻¹(70/300)
θ = 13.13° west of north.