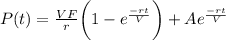Question

Pollution in a Lake. Consider a lake with a stream flowing out of it and a nearby factory dumping pollution into it. Without worrying about the modeling details yet, the mass of pollution in the lake is represented by dP dt = − r/V P + F, where P is the mass of pollution in the lake (kg), r is the flow rate of the stream (m3/sec), V is the volume of the lake (m3) and F is the mass of pollution dumped per time by the factory (kg/sec). Treat r, V and F as constants. Use an integrating factor to solve the ODE in general form. Find the constant of integration if the initial condition is P(0) = A, and write the solution for P(t) in a clean, final form.

Answer 1

`P(t) = \displaysyle(VF)/(r)\bigg(1 - e^{(-rt)/(V)}\bigg) + Ae^{(-rt)/(V)}`

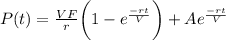
Explanation:

We are given that pollution in a lake is given by the differential equation:

`\displaystyle(dP(t))/(dt) = -\displaystyle(r)/(V)P(t) + F`

where, P(t) is the pollution at time t, r is the flow rate, V is the volume of lake and F is the mass of pollution dumped.

The given differential equation can be written as:

`\displaystyle(dP(t))/(dt) + \displaystyle(r)/(V)P(t) = F`

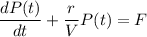
Comparing to linear differential equation:

`\displaystyle(dP(t))/(dt) = a(t)P(t) + b(t)`,

we get,

`a(t) = \displaystyle(r)/(V), b(t) = F`

Integrating factor:

`e^(\int a(t)dt) = e^{(r)/(V)dt} = e^{(rt)/(V)}`

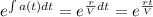
Solution:

`P(t)\text{Integrating Factor} = \int b(t)\text{Integrating Factor} + C\\\\P(t)e^{(rt)/(V)}= \int Fe^{(rt)/(V)}dt + C`

`P(t)e^{(rt)/(V)}= \displaysyle(VF)/(r)e^{(rt)/(V)} + C`,

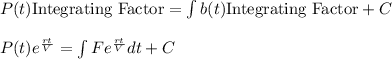
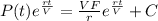where C is the constant of integration.

Now, we are given that P(0) = A

putting these value in the above equation, we get,

`A = \displaystyle(VF)/(r) + C\\\\C = A - \displaystyle(VF)/(r)`

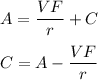
Putting this value of C in equation, we get:

`P(t)e^{(rt)/(V)} = \displaysyle(VF)/(r)e^{(rt)/(V)} + A - \displaystyle(VF)/(r)`

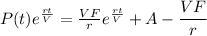
Dividing the equation by
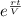`e^{(rt)/(V)}`, we get:

`P(t) = \displaysyle(VF)/(r) + \bigg(A - \displaystyle(VF)/(r)\bigg)e^{(-rt)/(V)}`

`P(t) = \displaysyle(VF)/(r)\bigg(1 - e^{(-rt)/(V)}\bigg) + Ae^{(-rt)/(V)}`