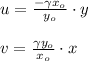Question

In a steady two-dimensional flow, particle trajectories are given byx(t) =x0cosγt;y(t) =y0sinγta. From these trajectories determine the Lagrangian particle velocity componentsu(t) =dx/dtandv(t) =dy/dt. Convert these to Eulerian velocity componentsu(x,y) andv(x,y). Note that the Eulerian velocity does not depend on time.

Answer 1

Answer with Explanation:

Given that

`x(t)=x_ocos(\gamma t)............(i)`

`y(t)=y_osin(\gamma t)..............(ii)`

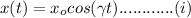
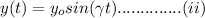
Thus by definition x component of velocity in Lagrangian system is given by

`u=(dx)/(dt)\\\\u=(d(x_ocos(\gamma t))/(dt)=-\gamma x_osin(\gamma t)`

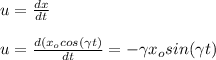
Thus by definition y component of velocity in Lagrangian system is given by

`v=(dy)/(dt)\\\\v=(d(y_osin(\gamma t))/(dt)=\gamma y_ocos(\gamma t)`

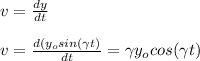
Since in eulerian system we need to eliminate time from the equations

From euations 'i' and 'ii' we can write

`cos(\gamma t)=(x)/(x_o)\\\\sin(\gamma t)=(y)/(y_o)`

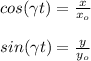
Applying these values in the velocity components as obtained in Lagrangian system we get

`u=(-\gamma x_o)/(y_o)\cdot y\\\\v=(\gamma y_o)/(x_o)\cdot x`