Question

Pls help me on this question :-

Answer 1

We conclude that the rule for the table in terms of x and y is:

• y = 3x+2

Explanation:

The table indicates that there is constant change in the x and y values, meaning the table represents the linear function the graph of which would be a straight line.

We know the slope-intercept form of the line equation

y = mx+b

where m is the slope and b is the y-intercept.

Taking two points

• (-2, -4)

• (-1, -1)

Finding the slope between (-2, -4) and (-1, -1)

`\mathrm{Slope}=(y_2-y_1)/(x_2-x_1)`

`\left(x_1,\:y_1\right)=\left(-2,\:-4\right),\:\left(x_2,\:y_2\right)=\left(-1,\:-1\right)`

`m=(-1-\left(-4\right))/(-1-\left(-2\right))`

`m=3`

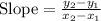
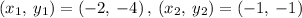
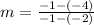
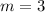
We know that the y-intercept can be determined by setting x = 0 and finding the corresponding y-value.

Taking another point (0, 2) from the table.

It means at x = 0, y = 2.

Thus, the y-intercept b = 2

Using the slope-intercept form of the linear line function

y = mx+b

substituting m = 3 and b = 2

y = 3x+2

Therefore, we conclude that the rule for the table in terms of x and y is:

• y = 3x+2