Question

What is the solution to –4(8 – 3x) ≥ 6x – 8?

x ≥ –x is greater than or equal to negative StartFraction 4 Over 3 EndFraction.
x ≤ –x is less than or equal to negative StartFraction 4 Over 3 EndFraction.
x ≥ 4
x ≤ 4

Answer 1

x ≥ 4

Explanation:

The given inequality is

`-4(8-3x)\geq6x-8`

Distribute -4 over the parenthesis

`-32+12x\geq6x-8`

Subtract 6x to both sides

`-32+6x\geq-8`

Add 32 to both sides

`6x\geq24`

Divide both sides by 6

`x\geq4`

Thus, the solution is x ≥ 4

Answer 2

`x\geq 4`

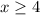
Explanation:

Let's start by using distributive property to get rid of the parenthesis on the left hand side of the inequality:

`-4(8-3x)\geq 6x-8\\-32+12x\geq 6x-8`

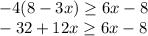
Now let's group all the terms that contain the variable x on the left, and all pure numerical terms on the right of the inequality symbol. To accomplish such, we subtract 6x from both sides, and then add 32 to both sides:

`-32+12x\geq 6x-8\\-32+12x-6x\geq -8\\-32+6x\geq -8\\6x\geq -8+32\\6x\geq 24`

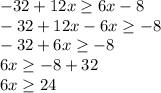
Now divide both sides of the inequality by positive 4 to isolate the "x" on one side:

`6x\geq 24\\x\geq (24)/(6) \\x\geq 4`

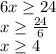
which agrees with the third option you listed.